Control of Underactuated Mechanical Systems

A mechanical system is called underactuated if the number of actuating inputs (applied forces or torques) is smaller than the number of mechanical degrees of freedom. If, on the other hand, the number of actuating interventions corresponds to the number of mechanical degrees of freedom, we speak of fully actuated systems. While the modeling of both system classes (underactuated or fully actuated) can be done in the same way with the common methods of rigid body mechanics (e.g. Newton, Euler-Langrange, Hamilton), underactuated systems are usually much more difficult to control than fully actuated systems. From a technical point of view, the transition to an underactuated system would correspond to the deliberate reduction of actuators and thus opens up an opportunity for material or cost savings. For special applications (e.g., in space technology), weight reduction could also be relevant. Likewise, it may be necessary to still be able to specifically influence certain systems in the event of an actuator defect.

Example: Planar Underactuated Manipulator

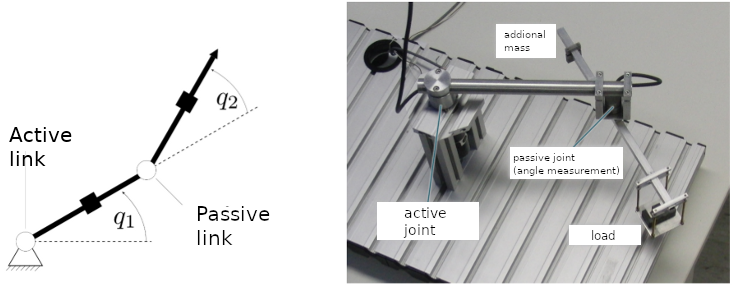
The next figure shows an underactuated two-link manipulator in the horizontal plane. The first link is active, second link is passive. The system’s input is the torque applied to the actuated link.

Publications

• Huang, C., Röbenack, K., & Knoll, C. (2016, March). Semi-analytic finite horizon control of an underactuated manipulator.
  In 2016 13th International Multi-Conference on Systems, Signals & Devices (SSD) (pp. 768-773). IEEE.
• Knoll, C.; Röbenack, K.: Maneuver Based Control of the 2-DOF Underactuated Manipulator in Normal Form Coordinates.
  Systems Science & Control Engineering, vol. 3, no. 1, 2014, 26-38.
• Knoll, C. & Röbenack, K.: Control of an underactuated manipulator using similarities to the double integrator.
  Preprints of the 18th IFAC World Congress, 2011, 11501-11507.
• Knoll, C.; Leist, B. & Röbenack, K.: Konzeption und prototypische Realisierung eines Versuchsstandes zur Regelung eines unteraktuierten Manipulators. In: Bertram, T.; Corves, B. & Janschek, K. (Hrsg.), Tagungsband Mechatronik 2011, 2011, 241-246.
• Knoll, C. & Röbenack, K.: Trajectory Planning for a Non Flat Mechanical System Using Time-Reversal Symmetry.
  Proc. in Applied Mathematics and Mechanics, 2011, 11, 819–820
• Knoll, C. & Röbenack, K.: Sliding Mode Control of an Underactuated Two-Link Manipulator.
  Proc. in Applied Mathematics and Mechanics, 2010, 10, 615-616.

Nonlinear Controllability and Brockett’s Condition

It is well known that underactuated mechanical systems without potential energy cannot be asymptotically stabilized to an equilibirum by a smooth time invariant feedback law due the Brockett’s condition. In [1] we give further necessary and sufficient conditions with respect to the Brockett’s condition and local controllability depending on the potential energy of the systems. In the paper [2] we show the global controllability of an underactuated two-link manipulator using the characteristic of the system’s drift vector field and weak Poisson stability.

Publications

1. Huang, C., & Röbenack, K. (2017, April). Brockett’s condition and local controllability in the context of underactuated mechanical systems.
   In 2017 3rd International Conference on Control, Automation and Robotics (ICCAR) (pp. 265-268). IEEE.
2. Huang, C., Röbenack, K., & Knoll, C. (2017). Nonlinear controllability of an underactuated two-link manipulator.
   International Journal of Digital Signals and Smart Systems, 1(3), 239-252.